Yuri Kivshar
【Link to comment】【Link to our work】
Metasurfaces, composed of an array of subwavelength optical scatterers on a surface, have demonstrated unprecedented capabilities of manipulating the properties of incoming light (e.g., amplitude, phase, polarization) [1], [2], [3]. Among the large family of metasurfaces, geometric metasurfaces have attracted great attention due to superior phase control [4], [5]. When circularly polarized light is illuminated and converted to the opposite handedness, geometric phase, also known as Pancharatnam-Berry (PB) phase, is locally introduced at each scatterer of the metasurface, whose value φ satisfies the simple relation of . Here, θ is the rotation angle of a scatterer, and σ takes the value of +1 and −1 for right- and left-handed circular polarization (RCP and LCP) incidence, respectively. By artificially arranging the rotation angles of scatterers over the metasurface, an arbitrary phase front can be generated, which has been widely applied for beam steering, structured light beam generation, hologram display, etc.
Up to now, geometric metasurfaces have been designed and operated under the assumption of locality, which means every scatterer is regarded as an independent unit and their mutual coupling is ignored. Such an assumption is only valid when the extended mode supported by many adjacent units is absent. In sharp contrast to local metasurfaces, nonlocal metasurfaces, also known as resonant metasurfaces, have recently been developed [6], [7], [8], [9]. Resonant metasurfaces can be harnessed for enhancing and modulating the incoherent luminescent process that occurs within the near field of the metasurface. But in this case, the assumption of locality is no longer valid, and it remains a challenging issue how to impart geometric phase into resonant metasurfaces. The rotation of a single scatterer can not only induce a local phase change, but also influence the optical responses of adjacent scatterers and even the whole metasurface.
In a recent paper [10], a novel type of resonant metasurface has been presented to successfully impart spin-dependent geometric phase into bound states in the continuum (BICs) [11]. By cyclically rotating notched disks within the metasurface, the photonic band containing a BIC mode at the Γ point is folded and extended in the Brillouin Zone (BZ), resulting in a series of synthetic valleys Γv in the extended BZs (Fig. 1a). Once the resonant perovskite metasurface is optically pumped, the luminescent photons coupled to the BIC mode will carry spin-dependent geometric phase and then selectively radiate to opposite valleys according to their spins (Fig. 1a). This spin-valley-locked emission has never been demonstrated before, and it enables simultaneously a record-high circular polarization degree of 0.91, a small beam divergent angle down to 1.6° and large emission angles up to 41°. Both emission angles and polarizations can be tailored by the rotation angles inside a supercell (Fig. 1b).
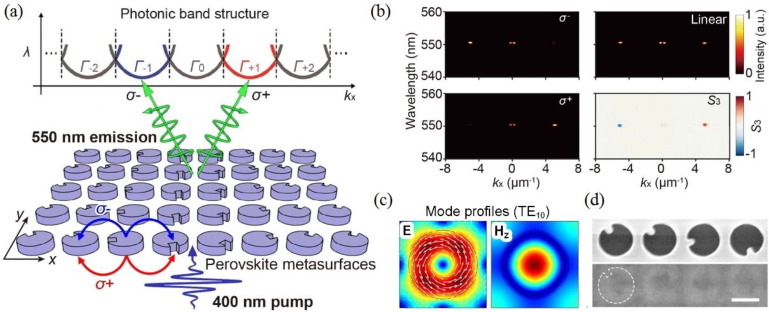
Fig. 1. (a) Schematic of the resonant geometric metasurface enabling spin-valley-locked perovskite emission. The Γ−1 (Γ+1) valley, formed by BZ folding, is selectively addressed by photon emission of σ− (σ+) polarization. (b) Measured spin-valley-locked lasing at the Γ±2 valleys. (c) Mode profiles of the TE10 BIC. (d) Scanning electron microscopy (SEM) images of the silica template (top) and perovskite metasurface (bottom). Scale bar: 200 nm. Figures are reproduced from Ref. [10].
For inducing geometric phase in resonant metasurfaces, the selection of resonant mode is important. In the proposed metasurface, although a series of Bloch modes can support BICs at the Γ point, the TE10 mode is intentionally selected, whose mode profiles represent a typical Mie resonance of out-of-plane magnetic dipole located at the center of the disk (Fig. 1c). Its corresponding electric fields exhibit an annular circulation around the periphery of the disk. As a result, the introduction of rotated notches will have similar perturbations to the BIC and still sustain it. Otherwise, the BIC mode may be collapsed by rotated notches. Furthermore, the TE10 mode is engineered to be the only BIC mode in the gain spectrum of perovskites, and its electric fields are mainly distributed inside the disks to be accessed by perovskites.
Another key point is the introduction of BZ folding for creating synthetic valleys. Synthetic valleys are mutually connected by the translation symmetry. They possess identical mode profiles and photonic density of state, and provide a versatile platform for inducing geometric phases. By arranging the rotation angles of units within a supercell, these valleys representing different radiation channels can be selectively addressed by emitted photons, as demonstrated in Ref. [10]. Although BZ folding has been known as an effective method for engineering photonic bands [12], here it is applied for geometric phase control.
Further, the theoretical method of analyzing and designing resonant metasurfaces is totally different from that of local metasurfaces. Each single scatterer cannot be simply regarded as an independent phase pixel. In this work, the emission property of the resonant metasurface is interpreted by tight-binding model, a semi-empirical method that is primarily used to calculate the electronic band structure of a material. The Hamiltonian of the metasurface is only approximately a sum of scatterer Hamiltonians located at different sites and scatterer wavefunctions overlap adjacent scatterer sites. The local scatterer mode is characterized by pseudospin-1 particles, and near-field coupling is described by the hopping and interaction of pseudospin-1 particles among the lattice sites. This theory has successfully predicted the spin-valley locking of metasurface emission. For an emitted photon to change valley, it has to flip its spin, leading to the robustness of both emission directionality and spin.
We note that the measured emission results match perfectly with theoretical predictions, which is largely attributed to the high-quality sample of perovskite metasurface. Usually, perovskite metasurfaces are fabricated by top-down methods of focused ion beam milling or reactive ion etching, resulting in the sample quality not high. Besides, the luminescent property of perovskites can be degraded during the process. But in this study, a unique fabrication method of self-limiting assembly is developed to allow the conformal growth of polycrystalline perovskites inside the template (Fig. 1d). A feature size down to 50 nm is well realized with long-range homogeneity. Therefore, the measured Q-factor is up to 700, leading to the low lasing threshold of 8.5 μJ/cm2.
In my view, this work represents an important breakthrough in the field of active metasurfaces. The resonant metasurfaces can modulate both coherent and incoherent emission processes, including thermoluminescence, electroluminescence, spontaneous parametric downconversion. Further, the concept of resonant geometric metasurface has the potential to be extended to more complex functionalities, such as focused emission, structured beam generation, and holographic emission.